Conservation of Energy EX 1

The car must have enough velocity at the top to go in a circle of radius $R$. When the car is at the top of the loop, its acceleration points downward and has the magnitude $v^{2} \over R$.

There are two forces acting on the car: $F_{G}$ pointing downward and the normal force $F_{N}$ exerted by the track which also points downward as shown in the free body diagram below:

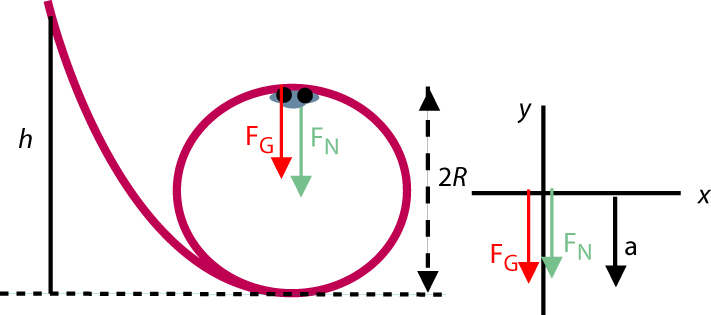
Writing the equation for the Newton's Second Law:

$-F_{G}-F_{N}=-m{v^{2} \over R}$

$F_{G}+F_{N}=m{v^{2} \over R}$

The magnitude of the gravitational force is given by the mass of the toy car. In the diagram above we have assumed that the car reaches to top of the loop. This may not be the case if the velocity is small enough so that

$F_{G}>m{v^{2} \over R}$

In such a case, the gravitational force is large enough to turn the car off the track. Thus, we have assumed by drawing the diagram as we did that the car reaches the top and that

$F_{G}\leqslant m{v^{2} \over R}$

We note that

• if $F_{G}>m{v^{2} \over R}\Rightarrow F_{N}=0$
• if $F_{G}\leqslant m{v^{2} \over R}\Rightarrow F_{N}>0$

Thus, the minimum speed the toy car needs to reach the top is given by

$mg=m{v^{2} \over R}$

$gR=v^{2}$

We can use the conservation of energy principle to determine the height of the car when it is released. We note that the work done by non-conservative forces is assumed to be zero ($W_{NC}=0$). The car is released from the rest ($K_{i}=0$) and it moves with the speed that we have computed above when it reaches the top of the track. Thus, the change in kinetic energy is

$\Delta K=K_{f}-K_{i}={\frac {1}{2}}mv^{2}-0={\frac {1}{2}}mgR$

Note that we have substituted the expression for the velocity in the above equation. The gravitational potential energy changes because the car moves from the initial height $h$ to the height $2R$ when the car is at the top of the loop. The change is

$\Delta U=U_{f}-U_{i}=mg(2R)-mgh$

We can use the law of conservation of energy

$\Delta K+\Delta U=W_{NC}$

We will substitute into this equation the previously computed values and solve

${\frac {1}{2}}mgR+2mgR-mgh=0$

$2.5R-h=0$

$2.5R=h$

The height $h=2.5R$ is the minimum height from which the car can be released and be expected to reach the top of the loop.