Conservative and Non-Conservative Forces

Kreshnik Angoni

CONSERVATIVE FORCES, POTENTIAL FUNCTION, SYSTEM : FORMAL DEFINITIONS

Some forces posses a specific quality: their work equals zero if the object on which they are exerted moves along a closed path.

These are known as conservative forces.

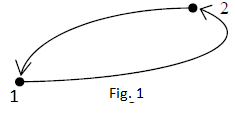
Note that a conservative force does a work different from zero when the object on which it acts is shifted from point “1” to point “2” (fig.1) but:

$W_{1-2}=-W_{2-1}\,\!$ so that $W_{1-1}=W_{1-2}+W_{2-1}=0\,\!$.

Three main examples of such forces are the gravitational, restoring (elastic) and static electric forces.

Not all the forces are conservative.

The net work by friction forces is negative (≠0) when object is shifted along a closed path.
The normal force is always perpendicular to displacement and its work is always zero, no matter what is the path.

Forces that depend on the velocity are not conservative
(the drag force in fluids, the magnetic force,..).

In the case of a conservative force, it can be concluded that:

If the object path is not closed, the work done by the force depends only on initial and final locations of object and not on the shape of the path to get from initial to final location.

One defines a potential U(x,y,z) or potential energy which is a function of space coordinates. This function is very useful for solving Physics problems.

Remember that a force is produced by its “source”.

If the force is conservative, one can use efficiently the concept of a "system" consisting of the “object that receives the force action AND the object (source) of this force” and then define the potential energy of the system.

Related Notes:

a) Any two or more objects experience their gravitational attraction forces. As gravitational forces are conservative, one may always define a system and a gravitational potential energy of this system.

If other conservative forces(ex. restoring forces) act between system’s components, one must add the corresponding terms to potential energy of the system.

(When the secondary forces are much smaller, at a first approximation, one may neglect them and consider only the main term of the potential).

b) The definition of a system cannot be tied to non-conservative forces because for them it is not possible to define a potential function. So, in mechanics, a non conservative force is an external force.

INTERNAL FORCES, TOTAL MECHANIAL ENERGY OF AN ISOLATED SYSTEM

-If one defines a system in upper terms, the source of the considered conservative force is part of the system and one deal with an internal force of this system. (If the “system” contains several “sources” and “objects”, the potential function will depend on a set of coordinates and there will be several internal forces.) If the system contains one “source” and one “object”, we fix the frame origin on the “source” and select Ox axe along the direction “source - object”. In this case, the potential function depends on xcoordinate and the force acting on the object, which is an internal force of the system, can be derived from the potential function U as:

$f=-{\frac {dU}{dx}}\,\!$ ............. (6)

The U- function (potential energy) is a system characteristic and it is often denoted Ep.

Exemple: An aeroplane at high h from earth surface. The gravitational force acting on the plane (weight) is a conservative force. The source of the weight is the earth. We define the system earth-aeroplane. We select Ox-axe along vertical direction and origin O is at earth center.

The potential energy of this system (we speak about aeroplane potential energy) is:

$U=E_{p}=m_{a}*g*x\,\!$  

Then, the plane weight ( internal force of system) can be calculated by use of formula:

$f_{x}=-{\frac {dU}{dx}}=-{\frac {d(m_{a}*g*x)}{dx}}=-m_{a}*g$ 

The “–“ shows the weight direction opposite to selected Ox positive direction.

-If a single conservative force is applied on the object of study, one may define a potential U, a system, tie a reference frame Ox to the other component of the system (source of force), calculate the kinetic energy of the object K (with respect to this frame), apply the work-energy theorem and find that the “sum of kinetic energy K plus potential energy U of the object remains constant in time” as follows:

$W_{net}=K_{fin}-K{in}=\Delta K\,\!$

For a small shift “dx” it will be produced a small work:

$dW_{net}=dK\,\!$ ........ (7)

As $dW_{net}=dW_{int}+dW_{ext}=dW_{int}\,\!$ (because $dW_{ext}=0\,\!$) and $dW_{int}=f_{int}*dx\,\!$ we get:

$dW_{net}=dW_{int}=f_{int}*dx=-{\frac {dU}{dx}}*dx=-dU=dK$

$\Rightarrow dK+dU=0$ 

$\Rightarrow K+U=CONST$ ........ (8) 

The potential energy concerns the object and the “source” of force.

As the kinetic energy of the “source” is zero (reference frame is tied to it), it comes out that the kinetic energy of the system is equal to that of the object under study.

So, actually the sum K + U presents the total mechanical energy of the system $E_{mech}$ and the expression (8) tells that

$E_{mech}=K+U=CONST\,\!$ ........ (9) 

Remember that we assumed no action from outside, i.e. an isolated system. So, the expression (9) shows that the total mechanical energy of an isolated system is conserved.

CONSERVATION OF MECANICAL ENERGY FOR NON ISOLATED SYSTEMS

How to deal with situations where any kind of forces (conservative and non-conservative) act on the same object while it is shifted from location “1” to location “2”?

1. One identify the conservative forces acting on the object under study.

2. One defines a common U potential for these forces and a common system.

3. One divides the set of all acting forces on the object into internal (that do contribute to U) and external (do not contribute to system potential function).

4. One notes that if there are no external forces the total mechanical energy of the system.
remains constant; $E_{mech-fin}=E_{mech-in}\,\!$.

So, if the external forces are doing a positive work $W_{ext-net}\,\!$ on the system (actually on “the object” which is part of the system) the total energy of the system will be increased by this amount and

$E_{mech-fin}=E_{mech-in}+W_{ext-net}\,\!$ or

$\Delta E_{mech}=E_{2-mech}-E_{1-mech}=W_{ext-net}\,\!$ ......... (10)

Notes: - The relation (10) is valid even for the case of a negative work by external forces. In this case it shows a decrease of total mechanical energy of the system.

- Expression (10) presents the general form of the principle of mechanical energy conservation. It is valid for any kind of system (isolated $W_{ext-net}=0\,\!$ or not isolated $W_{ext-net}\,\!$ ≠ 0).

REMEMBER: The kinetic, potential and total mechanical energies of a system are mathematical functions defined at a reference frame.

‐ While the definition of kinetic energy does not need the concept of system, potential and total energy functions cannot be defined without going through system concept.

The value of any of functions (K, U, E) by itself does not have a precise physical meaning for the system because they depend on the reference frame choice, too.

A simple change of the reference frame changes the value of those functions. But, the change of those functions has a very precise physical meaning. It is related to the achieved work and this quantity does not depend on the selected frame for calculations.

This is the basic issue one must not forget when dealing with energy related problems. The zero value of energy function can be selected following the convenience of problem requirements but the difference between its values must equal the net external work done on the system.

COMPARISON OF CONSERVATIVE AND NON-CONSERVATIVE FORCES

Helena Dedic

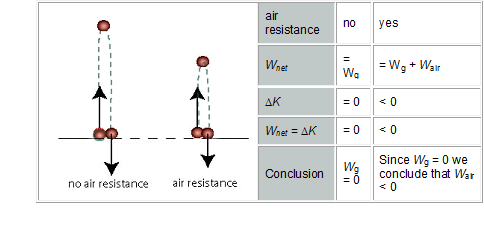
Comparison of the work done on a closed path (a closed path begins and ends at the same point) by the gravitational force and by the force of air resistance: a vertical flight of a ball with and without air resistance

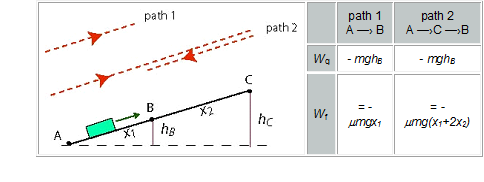
Comparison of path dependence of work done by gravity and by friction for block sliding on incline

path 1: from A directly to B, path 2: from A to B via C

• Conclusion: The work done by gravity is indpendent of the path while the work done be friction depends on the path.

Conservative forces

o $W_{c}\,\!$ , the work done by a conservative force, around any closed path is zero
o $W_{C}\,\!$ , the work done by a conservative force is independent of the path taken
o Examples: gravitational force , spring force

Non-conservative forces

o $W_{NC}\,\!$ , the work done by a non-conservative force around any closed path is different than zero

o $W_{NC}\,\!$ , the work done by a non-conservative force depends on the path taken

o Examples : resistive forces, friction, tension, applied forces

• Given the distinction between these two types of forces we will rewrite the statement of the work-kinetic energy theorem as follows:

$\Delta K=W_{C}+W_{NC}\,\!$ 

where $W_{C}\,\!$ is the sum of the work done by conservative forces and$W_{NC}\,\!$ is the sum of the work done by non-conservative forces.

EXERCISES

• Exercises: Conservative and Non-Conservative Forces