Newton's 3rd Law EX 3

The man pushes on the box with force of $F_{MB}=200$ N at 30° below the horizontal while the box pushes back on him with an equal and opposite force $F_{BM}=200$ N at 30° above the horizontal.

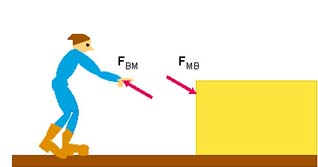
(a) The system we will consider now is the box.

The forces exerted on the box: $F_{G}$ $_{EB}$ (the gravitational force exerted by the Earth on the box), $F_{N}$ $_{FB}$ (the normal force exerted by the floor on the box), $F_{MB}$ (the force exerted by the man on the box), $F_{f}$ $_{FB}$ (the frictional force exerted by the floor on the box).

The box does not move and therefore the acceleration is zero. We will choose the coordinate system with x-axis parallel to the floor. The diagram below shows all the forces acting on the box and the appropriate free body diagram.

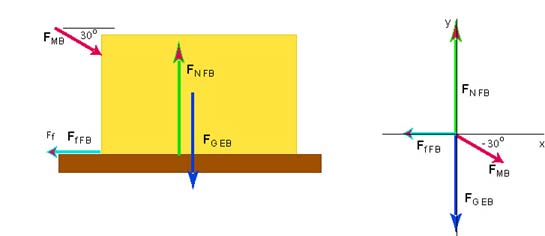
The components of these forces are shown in the table below:

Forces	x-component	y-component
$F_{G}$ $_{EB}$	0	$-mg=-(100\times 10)=-1000$ N
$F_{N}$ $_{FB}$	0	$F_{N}$ $_{FB}$
$F_{f}$ $_{FB}$	$-F_{f}$ $_{FB}$	0
$F_{MB}$	$F_{MB}\cos(-30^{\circ })=(200)(0.867)=173$ N	$F_{MB}\sin(-30^{\circ })=(200)(-0.5)=-100$ N

Now we use Newton's Second Law: $\Sigma F_{x}=0$ (no acceleration)

$-F_{f}$ $_{FB}+173=0$

We find that the friction force has a magnitude of 173 N.

Then we use $\Sigma F_{y}=0$

$-1000+F_{N}$ $_{FB}+(-100)=0$

We find that the normal force has a magnitude of 1100 N.

(b) The system we will consider now is the man.

The forces exerted on the man: $F_{G}$ $_{EM}$ (the gravitational force exerted by the Earth on the man), $F_{N}$ $_{FM}$ (the normal force exerted by the floor on the man), $F_{BM}$ (the force exerted by the box on the man), $F_{f}$ $_{FM}$ (the frictional force exerted by the floor on the man).

The man does not move and therefore the acceleration is zero. We will choose the coordinate system with x-axis parallel to the floor. The diagram below shows all the forces acting on the man and the appropriate free body diagram.

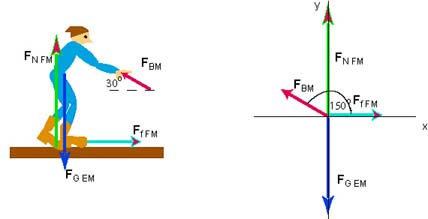
The components of these forces are shown in the table below:

Forces	x-component	y-component
$F_{G}$ $_{EM}$	0	$-mg=-(70\times 10)=-700$ N
$F_{N}$ $_{FM}$	0	$F_{N}$ $_{FM}$
$F_{f}$ $_{FM}$	$-F_{f}$ $_{FM}$	0
$F_{BM}$	$F_{BM}\cos(150^{\circ })=(200)(-0.867)=-173$ N	$F_{BM}\sin(150^{\circ })=(200)(0.5)=100$ N

Now we use Newton's Second Law: $\Sigma F_{x}=0$ (no acceleration)

$F_{f}$ $_{FM}+(-173)=0$

We find that the friction force has a magnitude of 173 N.

Then we use $\Sigma F_{y}=0$

$-700+F_{N}$ $_{FM}+100=600$

We find that the normal force has a magnitude of 600 N.